13 Mar Probability and Expectations
The sun is pretty likely to rise tomorrow – you can have confidence in that, but it is sometimes said that “there is no guarantee.” Scheduling meetings tomorrow based on the sunrise assumption is a safe bet, but there may be any number of other things that interfere with the meeting. Life is filled with risks, and in taking risks, remarkable opportunities.
Probability is an essential component of human reasoning. We use our experiences to infer probability and build expectations. Probability represents the mathematically quantifiable validity of human expectations. The source of these expectations may be rational or irrational, but we humans, whether consciously or otherwise, use probabilistic reasoning in almost all we do.
| Understanding Context Cross-Reference |
|---|
| Click on these Links to other posts and glossary/bibliography references |
|
|
|
|
| Prior Post | Next Post |
| Building a Concept Hierarchy | That’s so Random! |
| Definitions | References |
| Probability expectations | Kallenberg 2010 |
| reasoning experience | Tillman 2012 |
Probability and expectations work inside domains or contexts. The probability of a “ball” leading to a “walk” is greater in the context of baseball than in the context of high society night life, but possibly not by much, unless the “ball” is described as “grand”. Let us place ourselves in a scientific context and consider the probability that the sun will rise 
tomorrow. This probability is absolutely incalculable in the chosen context, because the sun’s relative position in the universe or galaxy is not defined in terms of up and down – we do not know if it has ever risen before because we do not know where up is. If we constrain the question a little more and attempt to determine the probability that the sun will rise over the Western Hemisphere tomorrow, forgiving imprecisions introduced by language and idiom, we can probably develop some assumptions and come up with an extremely large and reassuring factor. These same sorts of calculations can be applied to much more useful applications like the probability of precipitation or storms.
For example: In the context of the state of Pennsylvania, what is the probability that March will “go out like a lion” this year when it “came in like a lamb?” This type of equation may be a little more fuzzy (subjective) than, for example, asking whether the 31st of March will bring heavy precipitation to Philadelphia when the 1st of March was sunny. The way we reduce subjectivity, or the possibility of multiple conflicting interpretations, is to constrain the equation to the most clearly defined parameters possible. This context rigging makes the math easy, but there are occasions when it can’t be done.
Determining Likelihood
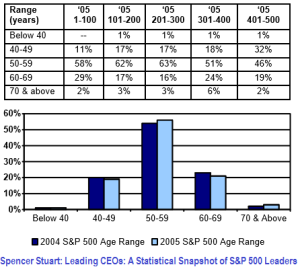Consider a two dimensional model to represent probability factors. In this model, we shall explore the probability that the Chief Executive Officer (CEO) of the S&P 500 company we are examining is within a certain age range. We have analyzed a list of the companies with the ages of their respective chiefs and plotted their frequencies on the bar graph at right. Supposing 290 of the chiefs were between 50 and 59 years old, if you picked any of the companies at random, there would be a 58% likelihood that the CEO was between 50 and 59 years old.
Although this example may not be extremely useful for you, personally, the science of determining probabilities is extremely practical for a variety of applications. Consider refinement of precious metals from ore. If you take a representative sample of ore and analyze its precious metal content, you can make a number of inferences regarding the probability of obtaining a profit from mining the tract in question if you know how much effort is required to extract the valuable components from the ore. You may even be able to predict costs and material requirements before beginning the mining operation. Probability theory is used every day in an enormous number of commercial applications.
Experience Linearity
Bound in time, as we are, we are forced to experience life one moment at a time. As we do, we are constantly predicting, sub consciously, what comes next. As a singer, I rely on this phenomenon to sight-read. Barbara Tillman has researched how: “Given the temporal nature of sound, expectations, structural integration, and cognitive sequencing are central in music perception (i.e., which sounds are most likely to come next and at what moment should they occur?)”.
Linear activities, including communication (I am typing one keystroke at a time at this moment), and other progressive sequential tasks involve lots of expectations, governed by learned probabilities. Whenever you cross a street, your brain makes a mental computation of the probability of safe passage. It’s the way our brains work. Fortunately, computers are very good at calculating probabilities quickly. Modeling this aspect of cognition is easy because it is so numerical and clearly defined.
| Click below to look in each Understanding Context section |
|---|
SHARE THIS ARTICLE
